You have now found out quite a lot about the customer's problem, and fluid flow in general. However, you still don't have a design to provide a range of flow-rates to your customer. You have observed that the pressure drop is proportional to flow-rate in the laminar regime, and proportional to the square of the flow-rate for turbulent flow. However, the flow-rates specified by the customer span the laminar, transitional, and turbulent regions. The transitional region is especially problematic because if you specify a certain pressure drop you are not sure what the flow-rate will be from one moment to the next!
Perhaps looking at the Reynolds number for this system will give you a way to design a reliable pumping system for the customer. Remember that the Reynolds number can be used to characterize flow in many different situations.
The Reynolds Number:
- D is the diameter of the pipe
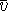is the average
velocity of the fluid, 
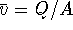, where A is the cross sectional area of the pipe
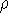is the density of the fluid
is the
viscosity of the fluid
- Re is a dimensionless number.
Previous Page . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . . Next Page